
Circuit Theory and Applications
Liver Disease Diagnosis using Tree-Based Machine Learning Algorithms
Liver Disease (LD) is a lethal yet relatively common disorder that is impacting the lives of millions across the world, causing slow yet irreversible internal organ damage or total organ failure if left untreated. In this study, LD is determined as the damage a Liver sustained due to excessive drug or alcohol abuse and other causes which leave severe scarring on the Liver leading to permanent functionality loss or cancer. This study revolves around determining if a person suffers from the disease regardless of the stage of the disease. To detect and minimize the impact of LD, tree-based
Threshold Energy Based LEACH-K Effect on the Accessibility of Wireless Sensor Networks
This paper aims to deliver an exhaustive investigation on the threshold energy parameter's direct impact on the Cluster Head (CH) selection phase in Low-Energy Adaptive Clustering Hierarchy Based on K-Means (LEACH-K) protocols. The most prominent threshold energy selection criterion out of the scarcely available research on the LEACH-K threshold energy parameter is used to simulate the LEACH-K protocol. Simulations are carried out on scaled-up Wireless Sensor Networks (WSNs) in terms of size and number of nodes. An analysis is performed on the life-cycle of the CH selection process, which
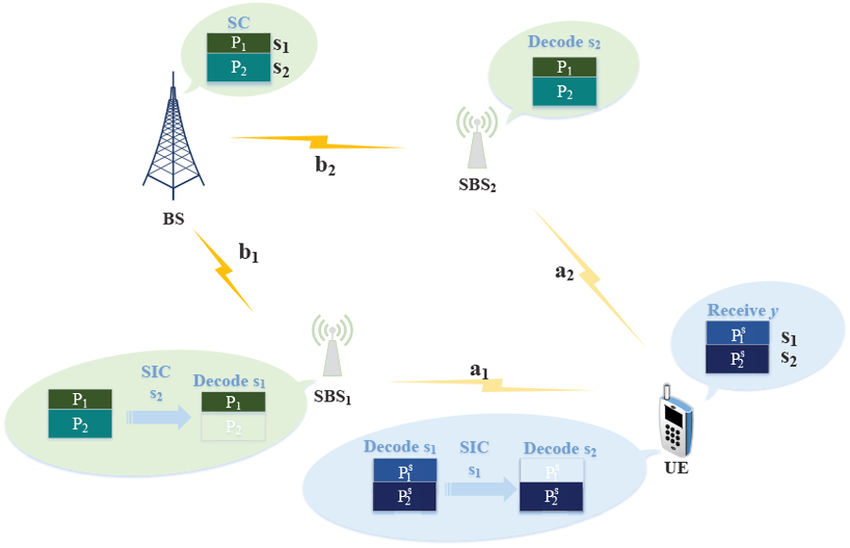
Relay Selection in NOMA-Based Cooperative Wireless Backhaul Networks
The joint application of wireless backhaul networks and non-orthogonal multiple access (NOMA) hold the potential to fulfill the increasing demands of fifth-generation (5G) communication networks and beyond. It is usual in wireless backhaul networks to take assistance from small cell base stations acting as intermediate relays to reach the remote destination. This cooperative communication is an acknowledged technique to combat multi-path fading, improve energy efficiency, and enhance the reliability and capacity of wireless networks. This article studies the application of relay selection (RS)

Novel Edge AI with Power-Efficient Re-configurable LP-MAC Processing Elements
Deep learning has become increasingly important in various fields, such as robotics, image processing, and speech recognition. However, the high computational requirements of deep learning models make it challenging to deploy them on edge & embedded devices with constrained power and area budgets. This paper proposes a novel low-power technique for implementing deep learning models on edge devices called LP-MAC (Low Power Multiply Accumulate). LP-MAC is designed for fixed-point format operations and takes advantage of reusing the input vector for MAC operations. It provides a new hardware

Sustainable Energy-Aware Task Scheduling for Wearable Medical Device Using Flower Pollination Algorithm
Power management and energy conservation are crucial for medical wearable devices that rely on energy harvesting. These devices operate under strict power budgets and require prolonged and stable operation. To achieve this, Energy-aware task scheduling is proposed as a solution to minimize energy consumption while ensuring the continued operational capabilities of the device. our paper presents a task scheduling method using the Flower Pollination Algorithm (FPA). The proposed task scheduling focuses on managing the activity of key components such as the heart rate sensor, temperature sensor

Modified Arnold Transform and DNA Manipulation for Chaos-Based RGB Image Encryption
Multimedia applications use image encryption algorithms extensively to safeguard and authenticate digital images. This paper presents an RGB image encryption method, which uses Chaos, DNA, pixel sum, and modified Arnold transform. The suggested algorithm is validated to be robust and resistant to visual, statistical, differential, and brute-force attacks. Additionally, the resulting encrypted images pass all tests of the NIST SP 800-22 test suite. © 2023 IEEE.
An Efficient DMO Task Scheduling Technique for Wearable Biomedical Devices
The popularity of wearable devices has grown as they improve the quality of life in many applications. In particular, for medical devices, energy harvesters are the dominating source of energy for wearable devices. However, their power budget is limited. Thus, power-saving techniques are essential components in the whole technology stack of those devices. That is, choosing the optimal schedule for different tasks running on the wearable device can help to reduce energy consumption. This paper presents a sensor task scheduling technique for optimizing energy consumption for energy harvesting

Implementation of Multi-Step Bias-Flip Rectifier for Piezoelectric Energy Harvesting
The full-wave rectifier is an essential step for extracting energy from a piezoelectric source. Yet, the inherent capacitance of the piezoelement significantly is considered a limitation of the efficiency of extraction. To address this issue, the bias-flip rectifier can be used. However, this rectifier needs large inductor and precise tuning. The large inductor increases the overall volume of the system which is inefficient. This paper address the problems with the traditional bias-flip rectifier by introducing an enhanced multi-step bias-flip rectifier to achieve a high voltage-flip
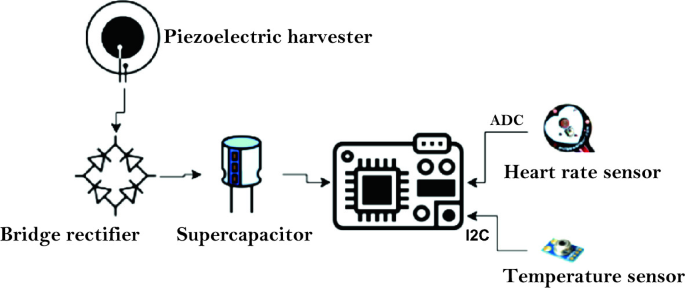
Energy Aware Tikhonov-Regularized FPA Technique for Task Scheduling in Wearable Biomedical Devices
Harvesting the energy from environmental sources is a promising solution for perpetual and continuous operation of biomedical wearable devices. Although the energy harvesting technology ensures the availability of energy source, yet power management is crucial to ensure prolonged and stable operation under a stringent power budget. Thus, power-aware task scheduling can play a key role in minimizing energy consumption to improve system durability while maintaining device functionality. This chapter proposes a novel biosensor task scheduling of energy harvesting-based biomedical wearable devices
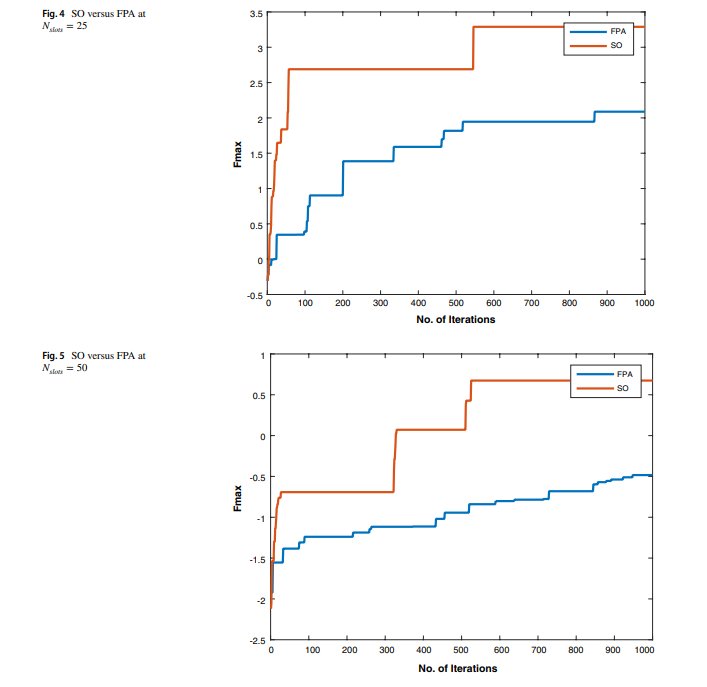
A power-aware task scheduler for energy harvesting-based wearable biomedical systems using snake optimizer
There is an increasing interest in energy harvesting for wearable biomedical devices. This requires power conservation and management to ensure long-term and steady operation. Hence, task scheduling algorithms will be used throughout this work to provide a reliable solution to minimize energy consumption while considering the system operation constraints. This study proposes a novel power-aware task scheduler to manage system operations. For example, we used the scheduler to handle system operations, including heart rate and temperature sensors. Two optimization techniques have been used to