This paper presents a generalization of well-known phase shift oscillator based on single CCII into the fractional order domain. The general state matrix, characteristic equation and design equations are presented. The general oscillation frequency, condition and the phase difference between the oscillatory outputs are introduced in terms of the fractional order parameters. These parameters add extra degrees of freedom which in turn increase the design flexibility and controllability. Numerical discussion of five special cases is investigated including the integer case. Spice simulations and

This paper proposes a hardware platform implementation on FPGA for two fractional-order derivative operators. The Grünwald-Letnikov and Caputo definitions are realized for different fractional orders. The realization is based on non-uniform segmentation algorithm with a variable lookup table. A generic implementation for Grünwald-Letnikov is proposed and a 32 bit Fixed Point Booth multiplier radix-4 is used for Caputo implementation. Carry look-ahead adder, multi-operand adder and booth multiplier are used to improve the performance and other techniques for area and delay minimization have

This paper presents a generalization of Soliman's four-phase oscillator into the fractional-order domain. The extra degrees of freedom provided by the fractional-order parameters α and β add more flexibility to the design of the circuit. The design procedure and equations of the proposed oscillator are presented and verified using Matlab and PSPICE. Also, the stability analysis for fractional order systems is studied for different cases of α and β. © 2017 IEEE.

Fractional-order calculus is the branch of mathematics which deals with non-integerorder differentiation and integration. Fractional calculus has recently found its way to engineering applications; particularly electronic circuits with promising results showing the feasibility of fabricating fractional-order capacitors on silicon. Fractionalorder capacitors are lossy non-deal capacitors with an impedance given by Zc = (1/jωC)α, where C is the pseudo-capacitance and α is its order (0

This paper presents a general procedure to obtain Butterworth filter specifications in the fractional-order domain where an infinite number of relationships could be obtained due to the extra independent fractional-order parameters which increase the filter degrees-of-freedom. The necessary and sufficient condition for achieving fractional-order Butterworth filter with a specific cutoff frequency is derived as a function of the orders in addition to the transfer function parameters. The effect of equal-orders on the filter bandwidth is discussed showing how the integer-order case is considered

This paper introduces new meta-heuristic optimization algorithms for extracting the parameters of the Cole-impedance model. It is one of the most important models providing best fitting with the measured data. The proposed algorithms inspired by nature are known as Flower Pollination Algorithm (FPA) and Moth-Flame Optimizer (MFO). The algorithms are tested over sets of both simulated and experimental data. The results are compared with other fitting algorithms such as the Non-linear least square (NLS) and Bacterial Foraging Optimization (BFO). The comparison showed a better fit in the sum of

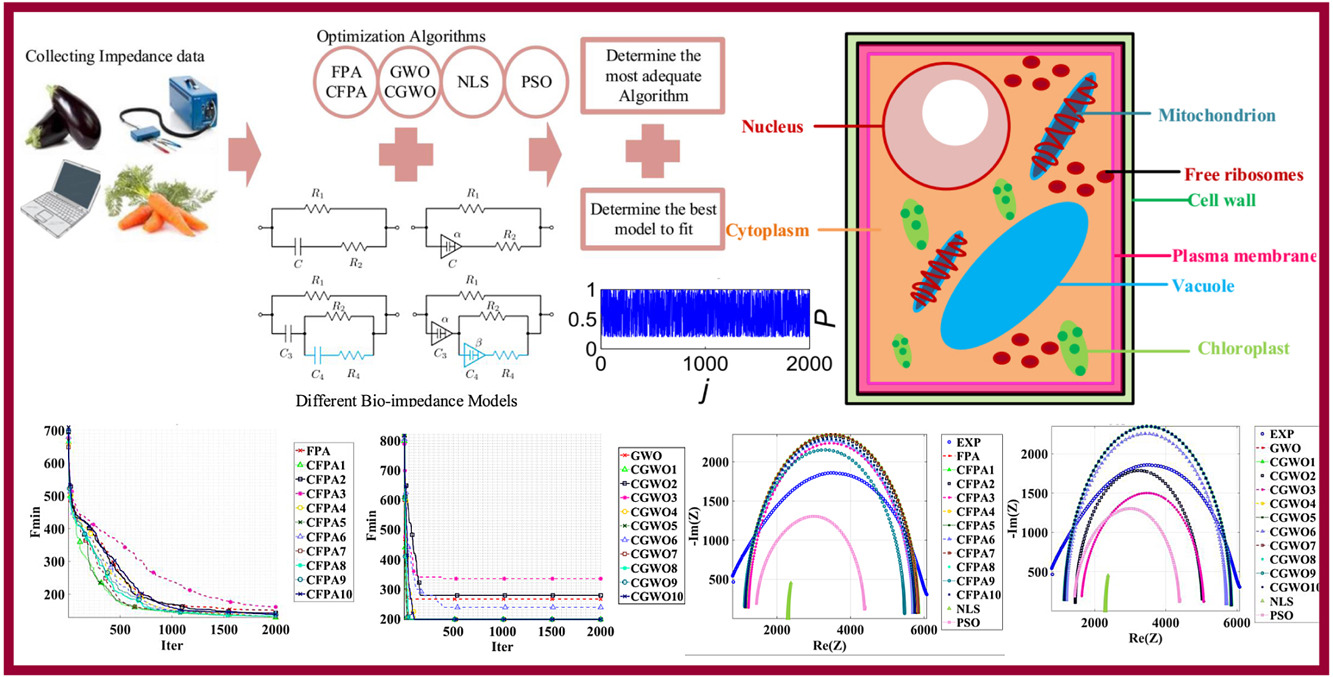
Precise parameter extraction of the bio-impedance models from the measured data is an important factor to evaluate the physiological changes of plant tissues. Traditional techniques employed in the literature for this problem are not robust which reflects on their accuracy. In this paper, the Flower Pollination Algorithm (FPA), the Grey Wolf Optimizer (GWO) and ten of their chaotic variants are employed to extract the parameters of bio-impedance models. These models are the simplified Hayden and Double-Shell models in their integer and fractional order forms. Experimental data sets of three

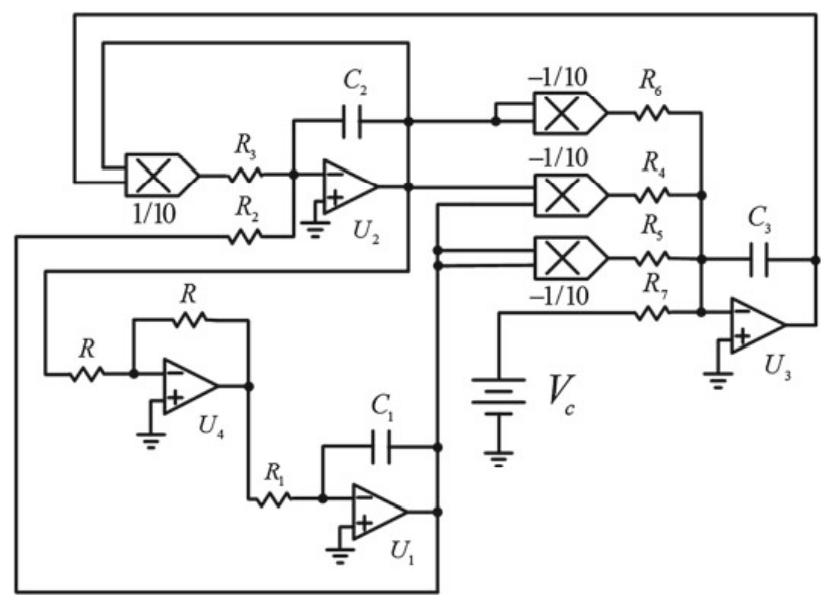
Recently, a new classification of nonlinear dynamics has been introduced by Leonov and Kuznetsov, in which two kinds of attractors are concentrated, i.e. self-excited and hidden ones. Self-excited attractor has a basin of attraction excited from unstable equilibria. So, from that point of view, most known systems, like Lorenz's system, Rössler's system, Chen's system, or Sprott's system, belong to chaotic systems with self-excited attractors. In contrast, a few unusual systems such as those with a line equilibrium, with stable equilibria, or without equilibrium, are classified into chaotic

Recently, a new classification of nonlinear dynamics has been introduced by Leonov and Kuznetsov, in which two kinds of attractors are concentrated, i.e. self-excited and hidden ones. Self-excited attractor has a basin of attraction excited from unstable equilibria. So, from that point of view, most known systems, like Lorenz’s system, Rössler’s system, Chen’s system, or Sprott’s system, belong to chaotic systems with self-excited attractors. In contrast, a few unusual systems such as those with a line equilibrium, with stable equilibria, or without equilibrium, are classified into chaotic

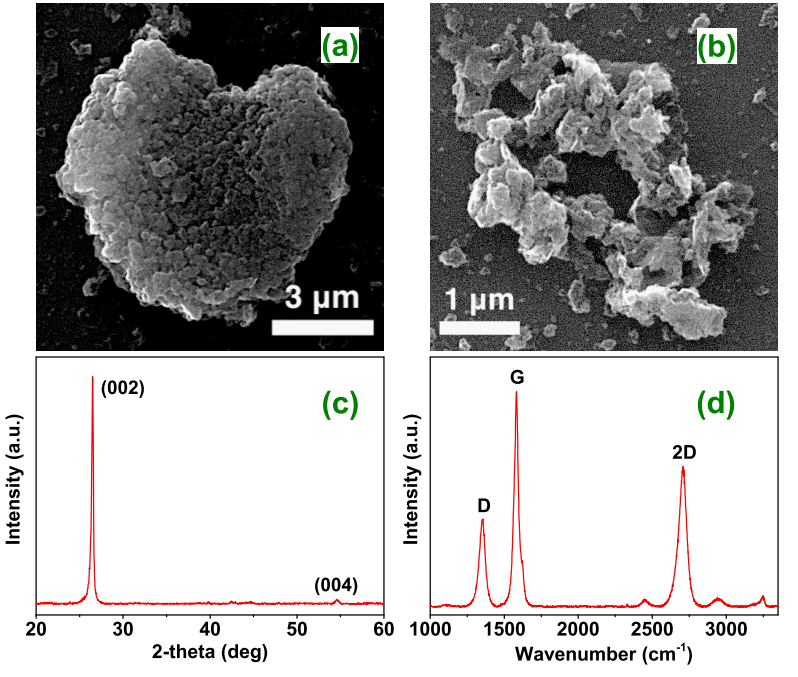
Supercapacitors are electrochemical devices that can store and restore electrical energy and are mostly used for powering dc or close-to-dc applications. As such they have not been explored enough for non-dc circuits. In this study, we implement a band-pass for frequency selectivity purposes and a relaxation oscillator for timing applications using a solid-state carbon electric double-layer capacitor. The expected behavior was observed for both circuits in the sub-Hertz and tens of Hertz frequency ranges. This confirms the possibility of using the frequency-dependent capacitive behavior of