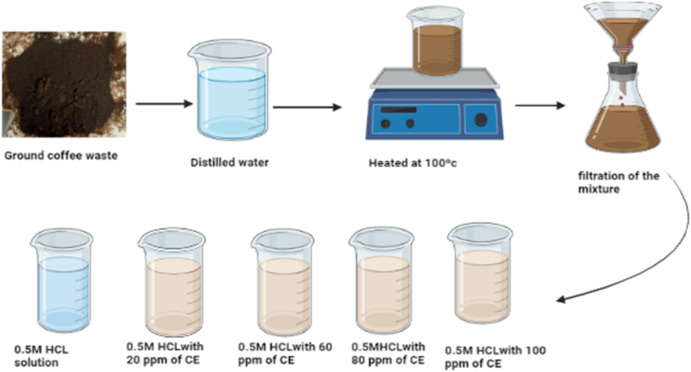
Corrosion poses a significant challenge in industrial sectors that handle metallic materials. The objective of this study was to evaluate the feasibility of employing a cost-effective, organic, and eco-friendly solution derived from discarded coffee grounds, known as coffee waste extract (CE), to mitigate the corrosion rate of carbon steel in an acidic environment comprising 0.5 M hydrochloric

Power management and energy conservation are crucial for medical wearable devices that rely on energy harvesting. These devices operate under strict power budgets and require prolonged and stable operation. To achieve this, Energy-aware task scheduling is proposed as a solution to minimize energy consumption while ensuring the continued operational capabilities of the device. our paper presents a

Retinal retinopathy of prematurity (ROP), an abnormal blood vessel formation, can occur in a baby who was born early or with a low birth weight. It is one of the primary causes of newborn blindness globally. Early detection of ROP is critical for slowing and stopping the progression of ROP-related vision impairment which leads to blindness. ROP is a relatively unknown condition, even among medical

All world countries are suffering from repercussions of the global climate change problems and there is an urgent need to mitigate their negative impacts. Improper waste management methods contribute directly to climate change where common waste treatment methods are basically relying on incineration or landfilling. Nowadays, incorporating the circular economy perspective into the business model

Indoor air pollution poses a substantial risk to human health and well-being, underscoring the crucial requirement for efficient monitoring systems. This paper introduces an advanced Air Pollution Monitoring System (APMS) tailored explicitly for indoor settings. The APMS integrates sensors and a user interface, ensuring the delivery of real-time and precise data concerning air quality parameters

Floating point representations are required in many applications due to their universality and ability to represent huge numbers accurately and in compact bit-width. Floating point arithmetic is complex, performance inefficient, and area-consuming compared to integer arithmetic operations. In this paper, hardware realization of area-efficient high-performance floating point arithmetic units for

Tactile memory is the cognitive process of storing and recalling information that has been perceived through the sense of touch. Directional tactile memory involves the encoding and retrieval of sensory data associated with a tactile experience, allowing individuals to remember and recognize directional information encoded through the sense of touch. A new method for providing directional tactile

TC21 alloy is a high-strength titanium alloy that has been gaining attention in various industries for its excellent combination of strength, toughness, and corrosion resistance. Given that this alloy is hard to cut material, therefore this study aims to optimize the process parameters of Turing this alloy under different conditions (i.e. as-received alloy, and heat-treated alloy). The L9 Taguchi

Modeling and simulation approach has been widely recommended for problem-solving and system enhancement in different industrial systems. The selection of the appropriate modeling technique and simulation tool is one of the most crucial parts of the simulation study as it affects the validity of the model and the reliability of the simulation. This study aims to provide a theoretical framework for

In terms of environmental and socio-economic benefits, the utilization of agro-fiber wastes in pulp-based product manufacturing is advantageous. Non-wood plant pulp production has shattered, and numerous non-wood fiber resources are being commercially used to make chemical pulp and paper in China, India, Latin America, Africa, the Middle East, and Turkey. Agricultural wastes, including rice and