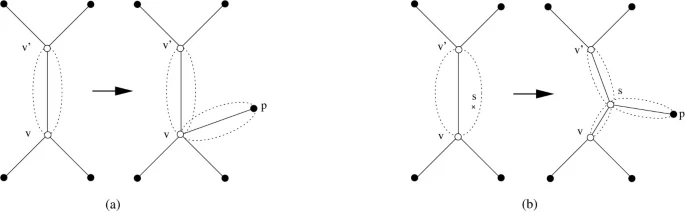
A fast algorithm for the multiple genome rearrangement problem with weighted reversals and transpositions

Background: Due to recent progress in genome sequencing, more and more data for phylogenetic reconstruction based on rearrangement distances between genomes become available. However, this phylogenetic reconstruction is a very challenging task. For the most simple distance measures (the breakpoint distance and the reversal distance), the problem is NP-hard even if one considers only three genomes. Results: In this paper, we present a new heuristic algorithm that directly constructs a phylogenetic tree w.r.t. the weighted reversal and transposition distance. Experimental results on previously published datasets show that constructing phylogenetic trees in this way results in better trees than constructing the trees w.r.t. the reversal distance, and recalculating the weight of the trees with the weighted reversal and transposition distance. An implementation of the algorithm can be obtained from the authors. Conclusion: The possibility of creating phylogenetic trees directly w.r.t. the weighted reversal and transposition distance results in biologically more realistic scenarios. Our algorithm can solve today's most challenging biological datasets in a reasonable amount of time. © 2008 Bader et al; licensee BioMed Central Ltd.