PRNG Using Primitive Roots of Primes and its Utilization in Chess-based Image Encryption
PRNG Using Primitive Roots of Primes and its Utilization in Chess-based Image Encryption
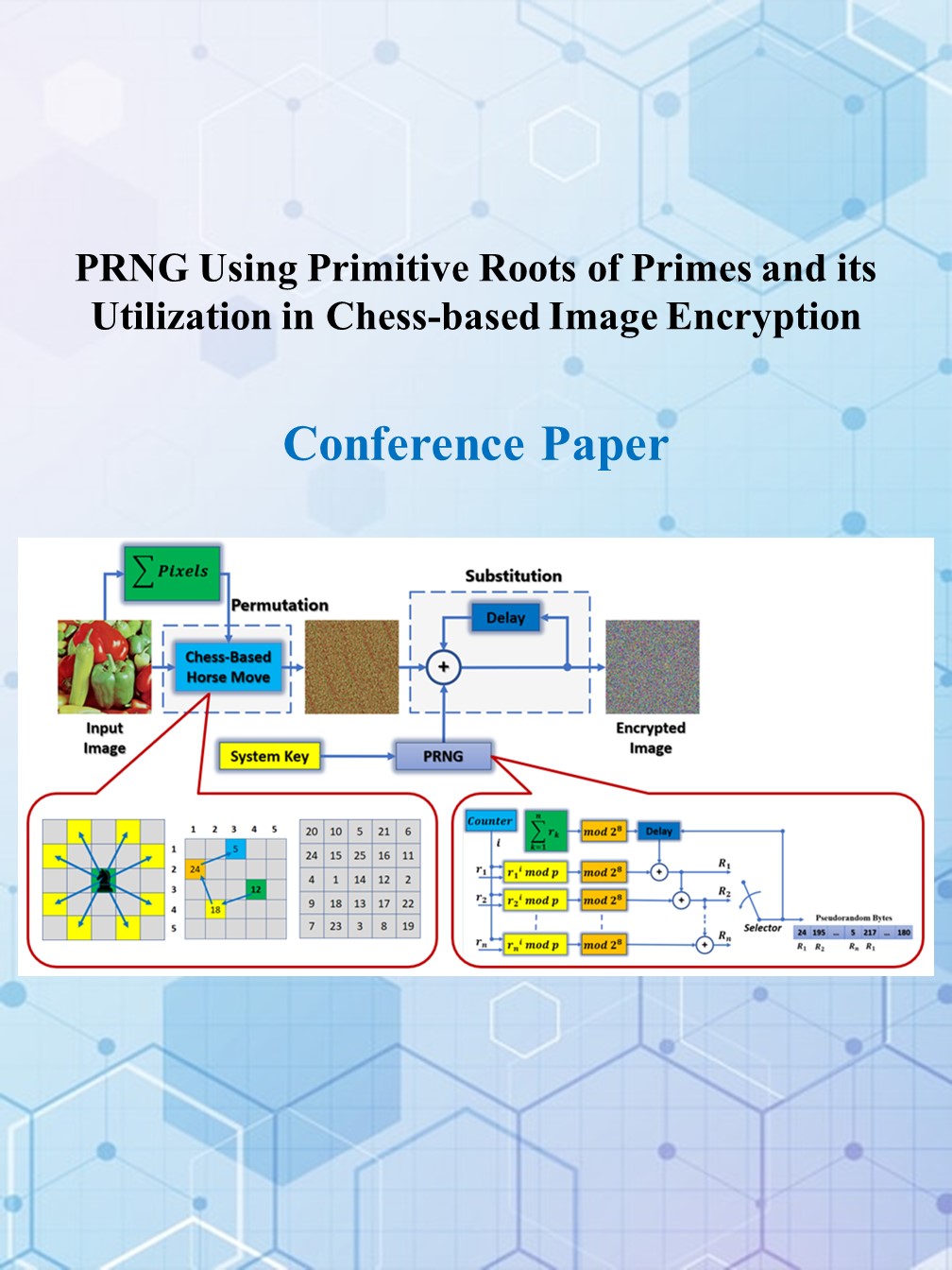
Recently, number theory has proved its importance in cryptography because of its well-known hard problems. For instance, a primitive root for a prime number shows a special property of uniqueness when raised to different powers mod the prime number. In this paper, a Pseudorandom Number Generator (PRNG) is designed based on this property using a prime number and some of its primitive roots. The PRNG is, first, validated for utilization in cryptography applications using histograms, correlation coefficients, and the National Institute of Standards and Technology (NIST) statistical test suite. Then, the PRNG is utilized in an image encryption system and the system security is tested using statistical measures, differential attack measures, and sensitivity to one-bit change. The results are promising and in the expected good ranges.
