The minimax approach for a class of variable order fractional differential equation
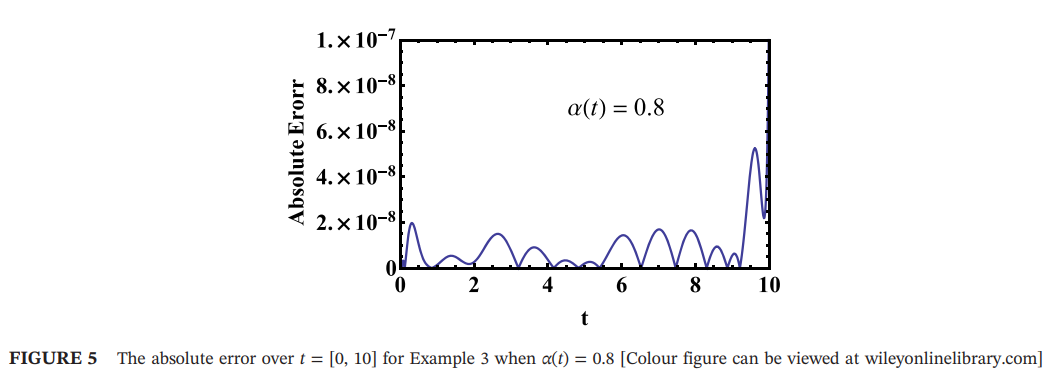
This paper introduces an approximate solution for Liouville-Caputo variable order fractional differential equations with order 0 < α(t) ≤ 1. The solution is adapted using a family of fractional-order Chebyshev functions with unknown coefficients. These coefficients have been obtained by using an optimization approach based on minimax technique and the least pth optimization function. Several linear and nonlinear fractional-order differential equations are discussed using the proposed technique for fixed and variable order fractional-order derivatives. Moreover, the response of RC charging circuit with variable order fractional capacitor is studied for different cases. Several comparisons with related published techniques have been added to illustrate the accuracy of the proposed approach. © 2019 John Wiley & Sons, Ltd.