Double-sided bifurcations in tent maps: Analysis and applications
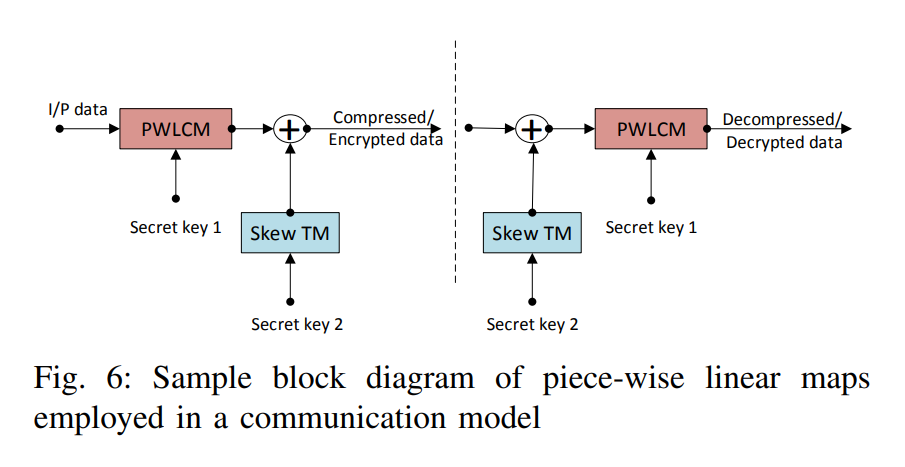
The tent map is a piece-wise linear one-dimensional discrete map which could be implemented easily. In this paper, a signed system parameter is allowed leading to the appearance of bidirectional bifurcations. A set of proposed tent maps with different sign variations and a signed parameter are investigated where the conventional map is a special case. The proposed maps exhibit period doubling as a route to chaos with wider and alternating sign output ranges that could fit multiple applications. Based on the maximum achievable output range corresponding to maximum chaotic behavior, the responses are called: positive tent map, mostly positive tent map, negative tent map, and mostly negative tent map. Mathematical analysis and results for the proposed maps are presented including: effective ranges of control parameter and iterated variable, key-points of the bifurcation diagram. Chaotic properties of the maps are explored including time series, cobweb diagrams, and maximum Lyapunov exponent. © 2016 IEEE.